POLYWARP Procedure

Standard Library procedure that calculates the coefficients needed for a polynomial image warping transformation.

Usage

POLYWARP, xd, yd, xin, yin, deg, xm, ym

Input Parameters

xd — The vector containing the x-coordinates to be fit as a function of (xin, yin).

yd — The vector containing the y-coordinates to be fit as a function of (xin, yin).

xin — The vector containing the independent x-coordinates. Must have the same number of points as xd.

yin — The vector containing the independent y-coordinates. Must have the same number of points as yd.

deg — The degree of the polynomial to be fitted to the data. The number of coordinate pairs formed by xin and yin must be greater than or equal to (deg + 1)².

Output Parameters

xm — A (deg + 1)-by-(deg + 1) array containing the coefficients of xd as a function of (xin, yin).

ym — A (deg + 1)-by-(deg + 1) array containing the coefficients of yd as a function of (xin, yin).

Keywords

None.

Discussion

POLYWARP calculates its transformation coefficients using the polynomial least-squares method. It returns the coefficients of the polynomial functions which best approximate the data:

The xm polynomial array pertains to the x direction.

The ym polynomial array pertains to the y direction.

POLYWARP determines the coefficients A_x(i, j) and A_y(i, j) of these two polynomial functions:

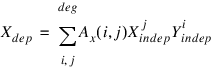
and:

where A_x = xm and A_y = ym.

Note:

The xm (x-coefficients) and ym (y-coefficients) can be used as input for the POLY_2D function. POLY_2D performs the actual warping of the image, using the x and y transformation coefficients that you provide.

Example

PRO polywarp_ex1

   ; Create a test image and display it.

   image = BYTSCL(DIST(300))

   WINDOW, XSize=300, YSize=300

   TV, image

   ; Create arrays describing four independent control points and

   ; plot these on top of image. These points describe a

   ; square, and represent the position of points in a

   ; calibration image.

   xin = [100, 200, 200, 100]

   yin = [200, 200, 100, 100]

   XYOUTS, 100, 200, '0', /Device

   XYOUTS, 200, 200, '1', /Device

   XYOUTS, 200, 100, '2', /Device

   XYOUTS, 100, 100, '3', /Device

   xd = INTARR(4)

   yd = INTARR(4)

   ; Pick four dependent warping control points from image;

   ; these points represent calibration points as actually

   ; measured by an instrument, which, due to distortion,

   ; has warped them.

   FOR i=0L,3 DO BEGIN

      PRINT, 'Pick warped point ', i

      CURSOR, xt, yt, /Device

      WAIT, 0.5

      xd(i) = xt

      yd(i) = yt

   ENDFOR

   deg = 1

   ; Perform linear (first degree) warping. POLYWARP returns

   ; xm and ym, the coefficients of the polynomial functions

   ; which describe this warping.

   POLYWARP, xd, yd, xin, yin, deg, xm, ym

   ; Apply the polynomial functions calculated with POLYWARP

   ; to the first image, using bilinear interpolation.

   interp = 1

   result = POLY_2D(image, xm, ym, interp)

   ; Display the resulting image, which represents the image

   ; after correcting for instrument distortion.

   TVSCL, result

END